Damped and Forced Oscillations
Equations of motion for damped and forced Oscillations
Damped Oscillations.
Consider the motion of a body in a viscous fluid in which the resistance to motion is proportional to the velocity. Suppose that the body has a weight W and that it is acted upon by a force- If k < n there is slight damping, so
- If k=n the motion is critically damped and there will be no oscillations. Both roots of the auxiliary equation are equal to -k.
- If k>n the result is a high degree of damping.
Forced Oscillations
If in addition to the forceExample:
[imperial]
Example - Damped Oscillation
Problem
A body which weighs 8 lbs. is acted upon by three forces
 and
and  ft/sec at
ft/sec at  find x in terms of t when:
find x in terms of t when:
- 6.25x lb.wt acting towards x = 0.
- A constant force of 2.5 lbs.wt.
- A resistance of
.
- r = 0.7
- r = 2.5
- r = 6.5
Workings
By Newton's Second Law the equation of motion is:
Re-arranging
Let x = 0.4 + y (x = 0.4 is the equilibrium position)
First Case
- In the first case r = 0.7 so the above equation becomes:
The resulting auxiliary equation is:
from which
If
and
at
whereThe Period of the oscillations is:Had there been no damping the Period would have been :
Second Case
- In the second case r = 2.5 and the equation of motion is :
The auxiliary equation is
which has equal roots of -5 If
and
at
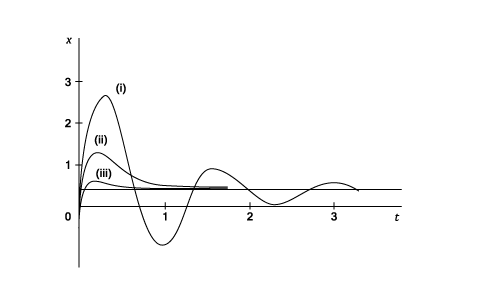
This has one maximum value of approximately 1.3 at t = 0.23 The body will pass through the equilibrium position of x = 0.4 at t = 0.4/14 and then creep back to it again as t tend to infinity.
Third Case
- In the third case r = 6.5 and the equations become:
and
which has roots m = -1 and m = -25
If
and
at
This has one maximum value
. The body passes through its equilibrium position when
and then creeps back to it as t tends to infinity. Had a smaller initial velocity been taken than 16 ft./sec. it would have been found that when r >2.5 the body would never reach its equilibrium position until t was infinite but that when r <2.5 it would oscillate just as before.
Solution
 Login
Login