Carnot Cycle

The ideal thermodynamic cycle

View version details

Contents

Overview
Thermal Efficiency
Page Comments

Key Facts

Gyroscopic Couple: The rate of change of angular momentum ( $\inline \tau$ ) = $\inline I\omega\Omega$ (In the limit).

$\inline I$ = Moment of Inertia.
$\inline \omega$ = Angular velocity
$\inline \Omega$ = Angular velocity of precession.

Overview

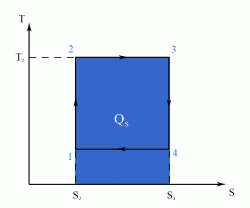
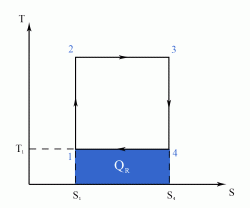
The Carnot Cycle is an entirely theoretical thermodynamic cycle utilising reversible processes.

The thermal efficiency of the cycle (and in general of any reversible cycle) represents the highest possible thermal efficiency (this statement is also known as Carnot's theorem - for a more detailed discussion see also Second Law of Thermodynamics ). This ultimate thermal efficiency can then be used to compare the efficiencies of other cycles operating between the same two temperatures.

The thermal efficiency of any engine working between the temperatures of T₁ and T₂ is:

$\eta_{th} < 1 - \frac{T_1}{T_2}$

(2)

From equation (2) it can be seen that in order to improve the thermal efficiency of an engine, we should basically increase the value of (T₂ - T₁), i.e. increase the temperature difference under which the engine works.

Thermal Efficiency

The thermal efficiency of a cycle, also denoted by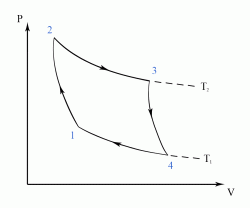 $\inline \eta_{th}$ , is a measure of the ability to convert heat energy into work. Therefore, the thermal efficiency can be defined as:

$\eta_{th} = \frac{W}{Q_S}$

(3)

where W is the work output, and Q_S the heat energy supplied. Replacing W with the heat supplied minus the head rejected, then equation (3) becomes:

$\eta_{th} = \frac{Q_S - Q_R}{Q_S}$

(4)

from which:

$\eta_{th} = 1 - \frac{Q_R}{Q_S}$

(5)

The cycle with the highest possible thermal efficiency is the Carnot cycle (diagramed on a $\inline PV$ plot in Figure 8).

MISSING IMAGE!

746/img_ther8.png cannot be found in /users/746/img_ther8.png. Please contact the submission author.

This cycle consists of

a reversible adiabatic (i.e. isentropic) compression of the gas from temperature T₁ to T₂ (step 1-2),
followed by an isothermal heating with expansion (step 2-3),
then a reversible adiabatic (isentropic) expansion of the gas from T₂ to T₁ (step 3-4),
and ending with an isothermal cooling with compression which reverts the system back to its initial state (step 4-1).

The Carnot Cycle can be represented on a TS diagram (see Figure 9), which is useful for calculating the Carnot Cycle efficiency.

MISSING IMAGE!

746/img_ther9-2.png cannot be found in /users/746/img_ther9-2.png. Please contact the submission author.

To calculate the thermal efficiency, first calculate the Q_S and Q_R terms (see Eq. 5). The heat supplied Q_S during step 2-3 can be calculated on a TS diagram as the area under the cycle beneath the T₂ line (the blue shaded area in Figure 9).

The area of this rectangle can also be calculated as:

$Q_S = T_2 (S_3 - S_2)$

(6)

On the other hand, the heat rejected Q_R during step 4-1 of the Carnot cycle can be calculated on a TS diagram as the area under the cycle beneath the T₁ line (the blue shaded area in Figure 9C).

MISSING IMAGE!

746/img_ther9-3.png cannot be found in /users/746/img_ther9-3.png. Please contact the submission author.

The area of this rectangle is also given by:

$Q_R = T_1 (S_4 - S_1)$

(7)

Taking into account (6) and (7), the thermal efficiency of the Carnot cycle becomes:

$\eta_{th} = 1 - \frac{T_1 (S_4 - S_1)}{T_2 (S_3 - S_2)}$

(8)

However, we can see from Figure 9A that $\inline S_1=S_2$ and $\inline S_3=S_4$ . Therefore, we obtain the Carnot cycle efficiency as:

$\eta_{th} = 1 - \frac{T_1}{T_2}$

(9)

or, written in a different form:

$\eta_{th} = \frac{T_2-T_1}{T_2}$

(10)

It should be noted that in equations (9) and (10) the temperatures (also identified as T_1=T_C, the temperature of the cold reservoir, and T_2=T_H, the temperature of the hot reservoir) are expressed on an absolute scale, such as the Kelvin scale. On the right side we provide calculators for the Carnot efficiency where you can input the temperatures in degrees Fahrenheit or degrees Celsius as well (the conversions are computed automatically).

Example:

[imperial]

Example - Heat supplied to a steam engine

Problem

Consider a steam engine for which the steam is supplied at $350^\circ F$ and condensed at $150^\circ F$ . If the thermal efficiency of the steam engine is $52\%$ of the Carnot efficiency, find the heat required (expressed in $BTU$ ) to produce a work output of 1 horsepower ( $HP$ ) for 1 minute.

Workings

We know that the Carnot efficiency of an engine working between temperatures $T_1$ and $T_2$ is given by:

$\eta_C = \frac{T_2-T_1}{T_2}$

(1)

where $T_1$ and $T_2$ are absolute temperatures expressed in kelvins ( $K$ ).

Therefore, in order to calculate the Carnot efficiency of the steam engine from the hypothesis, we first have to convert the temperatures into absolute temperatures. As we have that:

$T_1 = 150^\circ F = 338.70 \; K$

(2)

and

$T_2 = 350^\circ F = 449.82 \; K$

(3)

we get the Carnot efficiency of the steam engine:

$\eta_C = \frac{449.82 - 338.70}{449.82}$

(4)

from which we obtain:

$\eta_C = 0.247$

(5)

or, expressed as percentage:

$\eta_C = 24.7\%$

(6)

As the actual efficiency of the steam engine is $52\%$ of the Carnot efficiency, we can calculate the actual efficiency $\eta$ as:

$\eta = \frac{52}{100} \cdot 0.247$

(7)

from which we obtain:

$\eta = 0.128$

(8)

or, expressed as percentage:

$\eta = 12.8\%$

(9)

We know that the thermal efficiency of an engine is given by:

$\eta = \frac{W}{Q_S}$

(10)

where $W$ is the work output, and $Q_S$ the heat supplied. Therefore, the heat supplied $Q_S$ in order to produce a work output of $W$ , while working at an efficiency of $\eta$ can be written as:

$Q_S = \frac{W}{\eta}$

(11)

From the hypothesis we have that the required work output $W$ is $1\;HP$ for $60\;s$ . Taking into account that $1\;HP=550\;ft-lb/s$ , the work $W$ can also be expressed in $ft-lb$ as:

$W = 1 \cdot 550 \cdot 60$

(12)

from which we obtain:

$W = 33000 \; ft-lb$

(13)

By using (13) and (8) in equation (11), and also considering that $1\;BTU = 778 \; ft-lb$ , we get the heat required (expressed in $BTU$ ) to produce $1\;HP$ for $1\; min$ as:

$Q_S = \frac{33000}{0.128\cdot 778}$

(14)

from which we obtain:

Solution

$Q_S = 331.38 \; BTU$

(15)