Second Law of Thermodynamics

An introduction to the second law of thermodynamics, and discussing Carnot's theorem.

View other versions (2)

Contents

Overview
Carnot's Theorem
Page Comments

Key Facts

Gyroscopic Couple: The rate of change of angular momentum ( $\inline \tau$ ) = $\inline I\omega\Omega$ (In the limit).

$\inline I$ = Moment of Inertia.
$\inline \omega$ = Angular velocity
$\inline \Omega$ = Angular velocity of precession.

Overview

Definitions

Heat engine is any machine that can convert heat into work and vice versa.

Available energy is the proportion of heat supplied to a machine which can be converted into mechanical work. This proportion is found to be dependent on the temperature of the heat source, and also on the temperature at which heat is rejected.

<br/>

Key facts

The second law of thermodynamics states that heat can not be transferred from a body at a lower temperature to a body at a higher temperature without the application of energy from an external source.

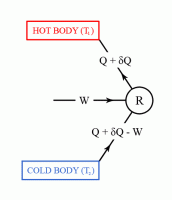
Carnot's theorem states that no machine can be more efficient than a machine working under a thermodynamically reversible cycle, provided that they are both working under the same limits of temperature.

Carnot's theorem can be proved by reductio ad absurdum using the second law of thermodynamics.

Carnot's Theorem

The second law of thermodynamics can be used to prove Carnot's theorem. However, before discussing Carnot's theorem, we first have to introduce the thermal efficiency of a heat engine.

The thermal efficiency of a heat engine, also denoted by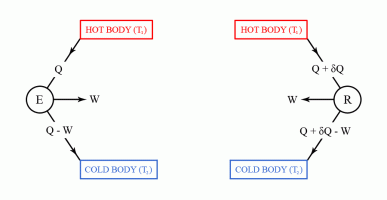 $\inline \eta_{th}$ , is a means of comparing the ability of an engine to convert heat energy into work. Therefore, the thermal efficiency can be defined as:

$\eta_{th} = \frac{W}{Q_S}$

(2)

where $\inline W$ is the mechanical work output, and $\inline Q_S$ the heat energy supplied.

As the first law of thermodynamics states that the energy output can not exceed the energy input (for a more detailed discussion see First Law of Thermodynamics ), we have:

$0 \leq \eta_{th} \leq 1$

(3)

or, expressed as percentages:

$0\% \leq \eta_{th} \leq 100\%$

(4)

Examples of thermal efficiencies are 4% for steam locomotives, 18%-30% for internal combustion engines, and 30% for modern power stations. From these examples it can be seen that the conversion of heat into work involves considerable losses in the available energy, and indeed, the efficiencies of most heat engines are generally much less than 100%.

In order to prove Carnot's theorem, consider two engines $\inline E$ and $\inline R$ - $\inline E$ working under an irreversible cycle, and $\inline R$ working under a reversible cycle. Also, assume by reductio ad absurdum that $\inline E$ is more efficient than $\inline R$ .

Consider that initially $\inline E$ takes $\inline Q$ units of heat at temperature $\inline T_1$ , and converts $\inline W$ into work, while $\inline (Q-W)$ units are rejected to a heat sink at temperature $\inline T_2$ (see Figure 1, left side). The thermal efficiency of engine $\inline E$ can thus be written as:

$\eta_E = \frac{W}{Q}$

(5)

At the same time, engine $\inline R$ takes $\inline (Q+\delta Q)$ units of heat at temperature $\inline T_1$ , and converts $\inline W$ into work, while $\inline (Q+\delta Q - W)$ units are rejected to a heat sink at temperature $\inline T_2$ (see Figure 1, right side). The thermal efficiency of engine $\inline R$ is thus given by:

$\eta_R = \frac{W}{Q+\delta Q}$

(6)

and as stated in the hypothesis, is less than that of engine $\inline E$ .

MISSING IMAGE!

746/img_thermo1.png cannot be found in /users/746/img_thermo1.png. Please contact the submission author.

We then consider the reverse process in the reversible engine $\inline R$ . Thus, $\inline R$ takes $\inline (Q+\delta Q - W)$ units of work to drive it backwards, and rejects $\inline (Q+\delta Q)$ units at temperature $\inline T_1$ (see Figure 2).

MISSING IMAGE!

746/img_thermo2.png cannot be found in /users/746/img_thermo2.png. Please contact the submission author.

Now imagine that the work output of engine $\inline E$ is used to provide the power to drive $\inline R$ backwards (see Figure 3).

MISSING IMAGE!

746/img_thermo3.png cannot be found in /users/746/img_thermo3.png. Please contact the submission author.

As can be seen from Figure 3, in these conditions $\inline \delta Q$ units of heat will be transferred from the cold sink to the hot sink. Considering that this is an isolated system within which no external work is being achieved, this would then contradict the second law of thermodynamics. Thus, the original assumption can not be true.

Therefore, indeed, there can not exist a machine more efficient than one working under a thermodynamically reversible cycle, provided that they are both working under the same limits of temperature (Carnot's theorem).